What Advances in Technology Science Medicine Math and Art Did Early Indian Society Produce
Information technology should come as no surprise that the first recorded utilise of the number cipher, recently discovered to be made equally early as the 3rd or 4th century, happened in India. Mathematics on the Indian subcontinent has a rich history going back over 3,000 years and thrived for centuries before similar advances were made in Europe, with its influence meanwhile spreading to China and the Eye East.
Also as giving united states of america the concept of aught, Indian mathematicians made seminal contributions to the study of trigonometry, algebra, arithmetic and negative numbers amid other areas. Perhaps most significantly, the decimal system that nosotros notwithstanding use worldwide today was start seen in Republic of india.
The number organisation
As far back equally 1200 BC, mathematical noesis was being written down as part of a big body of knowledge known as the Vedas. In these texts, numbers were commonly expressed as combinations of powers of ten. For instance, 365 might be expressed as three hundreds (3x10²), half dozen tens (6x10¹) and five units (5x10⁰), though each power of ten was represented with a name rather than a set of symbols. It is reasonable to believe that this representation using powers of 10 played a crucial role in the development of the decimal-place value system in India.
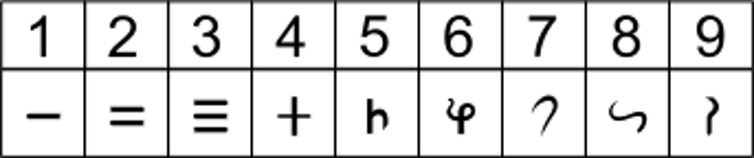
From the third century BC, we also accept written evidence of the Brahmi numerals, the precursors to the modernistic, Indian or Hindu-Standard arabic numeral arrangement that most of the world uses today. Once cypher was introduced, almost all of the mathematical mechanics would be in place to enable aboriginal Indians to study higher mathematics.
The concept of zero
Aught itself has a much longer history. The recently dated first recorded zeros, in what is known as the Bakhshali manuscript, were unproblematic placeholders – a tool to distinguish 100 from 10. Similar marks had already been seen in the Babylonian and Mayan cultures in the early centuries AD and arguably in Sumerian mathematics as early every bit 3000-2000 BC.
Merely but in India did the placeholder symbol for nothing progress to get a number in its ain right. The advent of the concept of zero immune numbers to exist written efficiently and reliably. In turn, this allowed for constructive record-keeping that meant important financial calculations could be checked retroactively, ensuring the honest actions of all involved. Cypher was a significant step on the route to the democratisation of mathematics.

These accessible mechanical tools for working with mathematical concepts, in combination with a strong and open up scholastic and scientific civilization, meant that, by around 600AD, all the ingredients were in identify for an explosion of mathematical discoveries in Bharat. In comparison, these sorts of tools were not popularised in the Due west until the early 13th century, though Fibonnacci's book liber abaci.
Solutions of quadratic equations
In the seventh century, the commencement written evidence of the rules for working with zero were formalised in the Brahmasputha Siddhanta. In his seminal text, the astronomer Brahmagupta introduced rules for solving quadratic equations (so beloved of secondary schoolhouse mathematics students) and for computing square roots.
Rules for negative numbers
Brahmagupta also demonstrated rules for working with negative numbers. He referred to positive numbers as fortunes and negative numbers as debts. He wrote downwardly rules that have been interpreted by translators as: "A fortune subtracted from naught is a debt," and "a debt subtracted from zero is a fortune".
This latter argument is the same every bit the rule nosotros learn in schoolhouse, that if yous subtract a negative number, it is the same equally adding a positive number. Brahmagupta also knew that "The product of a debt and a fortune is a debt" – a positive number multiplied past a negative is a negative.

For the large office, European mathematicians were reluctant to accept negative numbers as meaningful. Many took the view that negative numbers were absurd. They reasoned that numbers were developed for counting and questioned what yous could count with negative numbers. Indian and Chinese mathematicians recognised early on that one answer to this question was debts.
For example, in a primitive farming context, if one farmer owes some other farmer 7 cows, then effectively the offset farmer has -7 cows. If the starting time farmer goes out to purchase some animals to repay his debt, he has to buy 7 cows and give them to the second farmer in society to bring his cow tally back to 0. From and then on, every cow he buys goes to his positive total.
Basis for calculus
This reluctance to adopt negative numbers, and indeed null, held European mathematics back for many years. Gottfried Wilhelm Leibniz was one of the starting time Europeans to use zero and the negatives in a systematic manner in his evolution of calculus in the late 17th century. Calculus is used to measure rates of changes and is of import in almost every branch of science, notably underpinning many key discoveries in modern physics.

But Indian mathematician Bhāskara had already discovered many of Leibniz's ideas over 500 years earlier. Bhāskara, as well made major contributions to algebra, arithmetic, geometry and trigonometry. He provided many results, for case on the solutions of sure "Doiphantine" equations, that would not be rediscovered in Europe for centuries.
The Kerala schoolhouse of astronomy and mathematics, founded by Madhava of Sangamagrama in the 1300s, was responsible for many firsts in mathematics, including the apply of mathematical induction and some early on calculus-related results. Although no systematic rules for calculus were developed by the Kerala school, its proponents first conceived of many of the results that would afterward be repeated in Europe including Taylor series expansions, infinitessimals and differentiation.
The leap, fabricated in Bharat, that transformed zip from a simple placeholder to a number in its own right indicates the mathematically aware civilization that was flourishing on the subcontinent at a time when Europe was stuck in the dark ages. Although its reputation suffers from the Eurocentric bias, the subcontinent has a strong mathematical heritage, which it continues into the 21st century by providing key players at the forefront of every co-operative of mathematics.
Source: https://theconversation.com/five-ways-ancient-india-changed-the-world-with-maths-84332
0 Response to "What Advances in Technology Science Medicine Math and Art Did Early Indian Society Produce"
Post a Comment